- Raybet比分 提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
2007 AMC12B 真题及答案详细解析
2007 AMC 12 B 真题
答案详细解析请参考文末
Problem 1
Isabella's house has 3 bedrooms. Each bedroom is 12 feet long, 10 feet wide, and 8 feet high. Isabella must paint the walls of all the bedrooms. Doorways and windows, which will not be painted, occupy 60 square feet in each bedroom. How many square feet of walls must be painted?
![]()
Problem 2
A college student drove his compact car 120 miles home for the weekend and averaged 30 miles per gallon. On the return trip the student drove his parents' SUV and averaged only 20 miles per gallon. What was the average gas mileage, in miles per gallon, for the round trip?
![]()
Problem 3
The point ![]() is the center of the circle circumscribed about triangle
is the center of the circle circumscribed about triangle ![]() , with
, with ![]() and
and ![]() , as shown. What is the degree measure of
, as shown. What is the degree measure of ![]() ?
?

Problem 4
At Frank's Fruit Market, 3 bananas cost as much as 2 apples, and 6 apples cost as much as 4 oranges. How many oranges cost as much as 18 bananas?
![]()
Problem 5
The 2007 AMC 12 contests will be scored by awarding 6 points for each correct response, 0 points for each incorrect response, and 1.5 points for each problem left unanswered. After looking over the 25 problems, Sarah has decided to attempt the first 22 and leave the last 3 unanswered. How many of the first 22 problems must she solve correctly in order to score at least 100 points?
![]()
Problem 6
Triangle ![]() has side lengths
has side lengths ![]() ,
, ![]() , and
, and ![]() . Two bugs start simultaneously from
. Two bugs start simultaneously from ![]() and crawl along the sides of the triangle in opposite directions at the same speed. They meet at point
and crawl along the sides of the triangle in opposite directions at the same speed. They meet at point ![]() . What is
. What is ![]() ?
?
![]()
Problem 7
All sides of the convex pentagon ![]() are of equal length, and
are of equal length, and ![]() . What is the degree measure of
. What is the degree measure of ![]() ?
?
![]()
Problem 8
Tom's age is ![]() years, which is also the sum of the ages of his three children. His age
years, which is also the sum of the ages of his three children. His age ![]() years ago was twice the sum of their ages then. What is
years ago was twice the sum of their ages then. What is ![]() ?
?
![]()
Problem 9
A function ![]() has the property that
has the property that ![]() for all real numbers
for all real numbers ![]() . What is
. What is ![]() ?
?
![]()
Problem 10
Some boys and girls are having a car wash to raise money for a class trip to China. Initially ![]() % of the group are girls. Shortly thereafter two girls leave and two boys arrive, and then
% of the group are girls. Shortly thereafter two girls leave and two boys arrive, and then ![]() % of the group are girls. How many girls were initially in the group?
% of the group are girls. How many girls were initially in the group?
![]()
Problem 11
The angles of quadrilateral ![]() satisfy
satisfy ![]() . What is the degree measure of
. What is the degree measure of ![]() , rounded to the nearest whole number?
, rounded to the nearest whole number?
![]()
Problem 12
A teacher gave a test to a class in which ![]() of the students are juniors and
of the students are juniors and ![]() are seniors. The average score on the test was
are seniors. The average score on the test was ![]() . The juniors all received the same score, and the average score of the seniors was
. The juniors all received the same score, and the average score of the seniors was ![]() . What score did each of the juniors receive on the test?
. What score did each of the juniors receive on the test?
![]()
Problem 13
A traffic light runs repeatedly through the following cycle: green for ![]() seconds, then yellow for
seconds, then yellow for ![]() seconds, and then red for
seconds, and then red for ![]() seconds. Leah picks a random three-second time interval to watch the light. What is the probability that the color changes while she is watching?
seconds. Leah picks a random three-second time interval to watch the light. What is the probability that the color changes while she is watching?
![]()
Problem 14
Point ![]() is inside equilateral
is inside equilateral ![]() . Points
. Points ![]() ,
, ![]() , and
, and ![]() are the feet of the perpendiculars from
are the feet of the perpendiculars from ![]() to
to ![]() ,
, ![]() , and
, and ![]() , respectively. Given that
, respectively. Given that ![]() ,
, ![]() , and
, and ![]() , what is
, what is ![]() ?
?
![]()
Problem 15
The geometric series ![]() has a sum of
has a sum of ![]() , and the terms involving odd powers of
, and the terms involving odd powers of ![]() have a sum of
have a sum of ![]() . What is
. What is ![]() ?
?
![]()
Problem 16
Each face of a regular tetrahedron is painted either red, white, or blue. Two colorings are considered indistinguishable if two congruent tetrahedra with those colorings can be rotated so that their appearances are identical. How many distinguishable colorings are possible?
![]()
Problem 17
If ![]() is a nonzero integer and
is a nonzero integer and ![]() is a positive number such that
is a positive number such that ![]() , what is the median of the set
, what is the median of the set ![]() ?
?
![]()
Problem 18
Let ![]() ,
, ![]() , and
, and ![]() be digits with
be digits with ![]() . The three-digit integer
. The three-digit integer ![]() lies one third of the way from the square of a positive integer to the square of the next larger integer. The integer
lies one third of the way from the square of a positive integer to the square of the next larger integer. The integer ![]() lies two thirds of the way between the same two squares. What is
lies two thirds of the way between the same two squares. What is ![]() ?
?
![]()
Problem 19
Rhombus ![]() , with side length
, with side length ![]() , is rolled to form a cylinder of volume
, is rolled to form a cylinder of volume ![]() by taping
by taping ![]() to
to ![]() . What is
. What is ![]() ?
?
![]()
Problem 20
The parallelogram bounded by the lines ![]() ,
, ![]() ,
, ![]() , and
, and ![]() has area
has area ![]() . The parallelogram bounded by the lines
. The parallelogram bounded by the lines ![]() ,
, ![]() ,
, ![]() , and
, and ![]() has area
has area ![]() . Given that
. Given that ![]() ,
, ![]() ,
, ![]() , and
, and ![]() are positive integers, what is the smallest possible value of
are positive integers, what is the smallest possible value of ![]() ?
?
![]()
Problem 21
The first ![]() positive integers are each written in base
positive integers are each written in base ![]() . How many of these base-
. How many of these base-![]() representations are palindromes? (A palindrome is a number that reads the same forward and backward.)
representations are palindromes? (A palindrome is a number that reads the same forward and backward.)
![]()
Problem 22
Two particles move along the edges of equilateral ![]() in the direction
in the direction![]() starting simultaneously and moving at the same speed. One starts at
starting simultaneously and moving at the same speed. One starts at ![]() , and the other starts at the midpoint of
, and the other starts at the midpoint of ![]() . The midpoint of the line segment joining the two particles traces out a path that encloses a region
. The midpoint of the line segment joining the two particles traces out a path that encloses a region ![]() . What is the ratio of the area of
. What is the ratio of the area of ![]() to the area of
to the area of ![]() ?
?
![]()
Problem 23
How many non-congruent right triangles with positive integer leg lengths have areas that are numerically equal to ![]() times their perimeters?
times their perimeters?
![]()
Problem 24
How many pairs of positive integers ![]() are there such that
are there such that ![]() and
and![]() is an integer?
is an integer?
![]()
Problem 25
Points ![]() and
and ![]() are located in 3-dimensional space with
are located in 3-dimensional space with ![]() and
and ![]() . The plane of
. The plane of ![]() is parallel to
is parallel to ![]() . What is the area of
. What is the area of ![]() ?
?
![]()
<hr />
2007 AMC12 B 真题答案详细解析
- There are four walls in each bedroom, since she can't paint floors or ceilings. So we calculate the number of square feet of wall there is in one bedroom:
![[(12*8)+(12*8)+(10*8)+(10*8)-60=160+192-60=292]](https://latex.artofproblemsolving.com/8/9/6/8968b7516fa32a5a781b0cdf5a083081e7cccb62.png)
We have three bedrooms, so she must paint
![[292*3=876 Rightarrow fbox{(E)}]](https://latex.artofproblemsolving.com/9/0/7/907763e71968ad689eb1bda16caae6e454bebbf5.png)
square feet of wall. -
Solution 1
The trip was
 miles long and took
miles long and took  gallons. Therefore, the average mileage was
gallons. Therefore, the average mileage was 
Solution 2
Alternatively, we can use the harmonic mean to get




- 18 bananas cost the same as 12 apples, and 12 apples cost the same as 8 oranges, so 18 bananas cost the same as
 oranges.
oranges. - She must get at least
 points, and that can only be possible by answering at least
points, and that can only be possible by answering at least  questions correctly.
questions correctly. 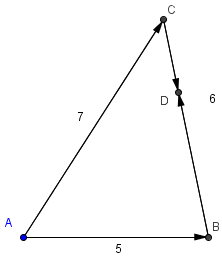 One bug goes to
One bug goes to  . The path that he takes is
. The path that he takes is  units long. The length of
units long. The length of  is
is 
 Since
Since  and
and  are right angles, and
are right angles, and  equals
equals  ,
,  is a square, and
is a square, and  is 5. Since
is 5. Since  and
and  are also 5, triangle
are also 5, triangle  is equilateral. Angle
is equilateral. Angle  is therefore
is therefore 


- The traffic light runs through a
 second cycle.Letting
second cycle.Letting  reference the moment it turns green, the light changes at three different times:
reference the moment it turns green, the light changes at three different times:  ,
,  , and
, and  This means that the light will change if the beginning of Leah's interval lies in
This means that the light will change if the beginning of Leah's interval lies in ![$[27,30]$](https://latex.artofproblemsolving.com/f/e/d/fed247ea7f8c97f0f1ded70f012592db772169a4.png) ,
, ![$[30,33]$](https://latex.artofproblemsolving.com/e/c/3/ec3d9f67ec329c02168e5579090fe14df62de947.png) or
or ![$[60,63]$](https://latex.artofproblemsolving.com/b/8/3/b83cfa74157bcba9f5ccdeaba2515a13d5bb7acd.png) This gives a total of
This gives a total of  seconds out of
seconds out of 

- Drawing
 ,
,  , and
, and  ,
,  is split into three smaller triangles. The altitudes of these triangles are given in the problem as
is split into three smaller triangles. The altitudes of these triangles are given in the problem as  ,
,  , and
, and  .Summing the areas of each of these triangles and equating it to the area of the entire triangle, we get:
.Summing the areas of each of these triangles and equating it to the area of the entire triangle, we get:![[frac{s(1)}{2} + frac{s(2)}{2} + frac{s(3)}{2} = frac{s^2sqrt{3}}{4}]](https://latex.artofproblemsolving.com/8/0/d/80daee549c2503b63654677de7eeac281db93a9a.png) where
where  is the length of a side
is the length of a side![[s = boxed{mathrm{(D) } 4sqrt{3}}]](https://latex.artofproblemsolving.com/d/d/d/ddd17ba78b8e87e6d688b7af272364fd4811013b.png)
- Note - This is called Viviani's Theorem on Wikipedia.
-
Solution 1
The sum of an infinite geometric series is given by
 where
where  is the first term and
is the first term and  is the common ratio.
is the common ratio.In this series,

The series with odd powers of
 is given as
is given as![[ar + ar^3 + ar^5 ...]](https://latex.artofproblemsolving.com/d/7/e/d7e66addf3f0977ed2667bd9ec0f8919e3857dfd.png)
It's sum can be given by

Doing a little algebra

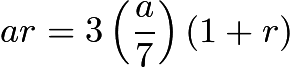




Solution 2
The given series can be decomposed as follows:

Clearly
 . We obtain that
. We obtain that  , hence
, hence  .
.Then from
 we get
we get  , and thus
, and thus  .
. - A tetrahedron has 4 sides. The ratio of the number of faces with each color must be one of the following:
 ,
,  ,
,  , or
, or  The first ratio yields
The first ratio yields  appearances, one of each color.The second ratio yields
appearances, one of each color.The second ratio yields  appearances, three choices for the first color, and two choices for the second.The third ratio yields
appearances, three choices for the first color, and two choices for the second.The third ratio yields  appearances since the two colors are interchangeable.The fourth ratio yields
appearances since the two colors are interchangeable.The fourth ratio yields  appearances. There are three choices for the first color, and since the second two colors are interchangeable, there is only one distinguishable pair that fits them.
appearances. There are three choices for the first color, and since the second two colors are interchangeable, there is only one distinguishable pair that fits them.
The total is
 appearances
appearances 
Solution 2
Every colouring can be represented in the form
 , where
, where  is the number of white faces,
is the number of white faces,  is the number of red faces, and
is the number of red faces, and  is the number of blue faces. Every distinguishable colouring pattern can be represented like this in exactly one way, and every ordered whole number triple with a total sum of 4 represents exactly one colouring pattern (if two tetrahedra have rearranged colours on their faces, it is always possible to rotate one so that it matches the other).
is the number of blue faces. Every distinguishable colouring pattern can be represented like this in exactly one way, and every ordered whole number triple with a total sum of 4 represents exactly one colouring pattern (if two tetrahedra have rearranged colours on their faces, it is always possible to rotate one so that it matches the other).Therefore, the number of colourings is equal to the number of ways 3 distinguishable nonnegative integers can add to 4. If you have 6 cockroaches in a row, this number is equal to the number of ways to pick two of the cockroaches to eat for dinner (because the remaining cockroaches in between are separated in to three sections with a non-negative number of cockroaches each), which is

- Note that if
 is positive, then, the equation will have no solutions for
is positive, then, the equation will have no solutions for  . This becomes more obvious by noting that at
. This becomes more obvious by noting that at  ,
,  . The LHS quadratic function will increase faster than the RHS logarithmic function, so they will never intersect.This puts
. The LHS quadratic function will increase faster than the RHS logarithmic function, so they will never intersect.This puts  as the smallest in the set since it must be negative.Checking the new equation:
as the smallest in the set since it must be negative.Checking the new equation:  Near
Near  ,
,  but at
but at  ,
,  This implies that the solution occurs somewhere in between:
This implies that the solution occurs somewhere in between:  This also implies that
This also implies that 
This makes our set (ordered)

The median is

Cheap Solution that most people probably used
Led
 . Then
. Then  giving
giving  . Then the ordered set is
. Then the ordered set is  and the median is
and the median is  so the answer is
so the answer is  .
. - The difference between
 and
and  is given by
is given by The difference between the two squares is three times this amount or
The difference between the two squares is three times this amount or The difference between two consecutive squares is always an odd number, therefore
The difference between two consecutive squares is always an odd number, therefore  is odd. We will show that
is odd. We will show that  must be 1. Otherwise we would be looking for two consecutive squares that are at least 81 apart. But already the equation
must be 1. Otherwise we would be looking for two consecutive squares that are at least 81 apart. But already the equation  solves to
solves to  , and
, and  has more than three digits.The consecutive squares with common difference
has more than three digits.The consecutive squares with common difference  are
are  and
and  . One third of the way between them is
. One third of the way between them is  and two thirds of the way is
and two thirds of the way is  .
.
This gives
 ,
,  ,
,  .
.
![[asy] pair B=(0,0), A=(6*dir(60)), C=(6,0); pair D=A+C; draw(A--B--C--D--A); draw(A--(3,0)); label("(A)",A,NW);label("(B)",B,SW);label("(C)",C,SE);label("(D)",D,NE); label("(6)",A/2,NW); label("(theta)",(.8,.5)); label("(h)",(3,2.6),E); [/asy]](https://latex.artofproblemsolving.com/d/4/2/d423bf62199e5f6372de91d9c23fe0f39077413b.png)
 Where
Where  and
and 




- Plotting the parallelogram on the coordinate plane, the 4 corners are at
 . Because
. Because  , we have that
, we have that  or that
or that  , which gives
, which gives  (consider a homothety, or dilation, that carries the first parallelogram to the second parallelogram; because the area increases by
(consider a homothety, or dilation, that carries the first parallelogram to the second parallelogram; because the area increases by  , it follows that the stretch along the diagonal, or the ratio of side lengths, is
, it follows that the stretch along the diagonal, or the ratio of side lengths, is  ). The area of triangular half of the parallelogram on the right side of the y-axis is given by
). The area of triangular half of the parallelogram on the right side of the y-axis is given by  , so substituting
, so substituting  :
:![[frac{1}{2} (c-d)left(frac{c-d}{a-b}right) = 9 quad Longrightarrow quad 2d^2 = 9(a-b)]](https://latex.artofproblemsolving.com/5/1/e/51edc5dfccf7d46f4df539eb3d687c0b4d8f4c04.png)
 , and we verify that
, and we verify that  ,
,  will give us a minimum value for
will give us a minimum value for  . Then
. Then  .
.
Solution 2
The key to this solution is that area is invariant under translation. By suitably shifting the plane, the problem is mapped to the lines
 and
and  . Now, the area of the parallelogram contained by is the former is equal to the area of a rectangle with sides
. Now, the area of the parallelogram contained by is the former is equal to the area of a rectangle with sides  and
and  ,
,  , and the area contained by the latter is
, and the area contained by the latter is  . Thus,
. Thus,  and
and  must be even if the former quantity is to equal
must be even if the former quantity is to equal  .
.  so
so  is a multiple of
is a multiple of  . Putting this all together, the minimal solution for
. Putting this all together, the minimal solution for  , so the sum is
, so the sum is  .
.  All numbers of six or less digits in base 3 have been written.The form of each palindrome is as follows1 digit -
All numbers of six or less digits in base 3 have been written.The form of each palindrome is as follows1 digit -  2 digits -
2 digits -  3 digits -
3 digits - 
4 digits -

5 digits -

6 digits -

Where
 are base 3 digits
are base 3 digitsSince
 , this gives a total of
, this gives a total of  palindromes so far.
palindromes so far.7 digits -
 , but not all of the numbers are less than
, but not all of the numbers are less than 
Case:

All of these numbers are less than
 giving
giving  more palindromes
more palindromesCase:
 ,
, 
All of these numbers are also small enough, giving
 more palindromes
more palindromesCase:
 ,
, 
It follows that
 , since any other
, since any other  would make the value too large. This leaves the number as
would make the value too large. This leaves the number as  . Checking each value of d, all of the three are small enough, so that gives
. Checking each value of d, all of the three are small enough, so that gives  more palindromes.
more palindromes.Summing our cases there are

- First, notice that each of the midpoints of
 ,
, , and
, and  are on the locus. Suppose after some time the particles have each been displaced by a short distance
are on the locus. Suppose after some time the particles have each been displaced by a short distance  , to new positions
, to new positions  and
and  respectively. Consider
respectively. Consider  and drop a perpendicular from
and drop a perpendicular from  to hit
to hit  at
at  . Then,
. Then,  and
and  . From here, we can use properties of a
. From here, we can use properties of a  triangle to determine the lengths
triangle to determine the lengths  and
and  as monomials in
as monomials in  . Thus, the locus of the midpoint will be linear between each of the three special points mentioned above. It follows that the locus consists of the only triangle with those three points as vertices. (A cheaper way to find the shape of the region is to look at the answer choices: if it were any sort of conic section then the ratio would not generally be rational.) Comparing inradii between this "midpoint" triangle and the original triangle, the area contained by
. Thus, the locus of the midpoint will be linear between each of the three special points mentioned above. It follows that the locus consists of the only triangle with those three points as vertices. (A cheaper way to find the shape of the region is to look at the answer choices: if it were any sort of conic section then the ratio would not generally be rational.) Comparing inradii between this "midpoint" triangle and the original triangle, the area contained by  must be
must be  of the total area.
of the total area. - Let
 and
and  be the two legs of the triangle.We have
be the two legs of the triangle.We have  .Then
.Then  .We can complete the square under the root, and we get,
.We can complete the square under the root, and we get,  .Let
.Let  and
and  , we have
, we have  .After rearranging, squaring both sides, and simplifying, we have
.After rearranging, squaring both sides, and simplifying, we have  .
.
Putting back
 and
and  , and after factoring using
, and after factoring using  , we've got
, we've got  .
.Factoring 72, we get 6 pairs of
 and
and 

And this gives us
 solutions
solutions  .
.Alternatively, note that
 . Then 72 has
. Then 72 has  factors. However, half of these are repeats, so we have
factors. However, half of these are repeats, so we have  solutions.
solutions.Solution #2
We will proceed by using the fact that
![$[ABC] = rcdot s$](https://latex.artofproblemsolving.com/2/8/9/289668c9ffccf64525a50cf707bd6a55f5edf196.png) , where
, where  is the radius of the incircle and
is the radius of the incircle and  is the semiperimeter
is the semiperimeter  .
.We are given
![$[ABC] = 3p = 6s Rightarrow rs = 6s Rightarrow r = 6$](https://latex.artofproblemsolving.com/6/f/a/6fa0b1a3f620e4e16086645fa3e07b02c60c7449.png) .
.The incircle of
 breaks the triangle's sides into segments such that
breaks the triangle's sides into segments such that  ,
,  and
and  . Since ABC is a right triangle, one of
. Since ABC is a right triangle, one of  ,
,  and
and  is equal to its radius, 6. Let's assume
is equal to its radius, 6. Let's assume  .
.The side lengths then become
 ,
,  and
and  . Plugging into Pythagorean's theorem:
. Plugging into Pythagorean's theorem:





We can factor
 to arrive with
to arrive with  pairs of solutions:
pairs of solutions:  and
and  .
. -
Solution 1
Combining the fraction,
 must be an integer.
must be an integer.Since the denominator contains a factor of
 ,
, 
Since
 for some positive integer
for some positive integer  , we can rewrite the fraction(divide by
, we can rewrite the fraction(divide by  on both top and bottom) as
on both top and bottom) as 
Since the denominator now contains a factor of
 , we get
, we get  .
.But since
 , we must have
, we must have  , and thus
, and thus  .
.For
 the original fraction simplifies to
the original fraction simplifies to  .
.For that to be an integer,
 must be a factor of
must be a factor of  , and therefore we must have
, and therefore we must have  . Each of these values does indeed yield an integer.
. Each of these values does indeed yield an integer.Thus there are four solutions:
 ,
,  ,
,  ,
,  and the answer is
and the answer is 
Solution 2
Let's assume that
 We get
We get
Factoring this, we get
 equations-
equations-



(It's all negative, because if we had positive signs,
 would be the opposite sign of
would be the opposite sign of  )
)Now we look at these, and see that-








This gives us
 solutions, but we note that the middle term needs to give you back
solutions, but we note that the middle term needs to give you back  .
.For example, in the case
 , the middle term is
, the middle term is  , which is not equal by
, which is not equal by  for any integer
for any integer  .
.Similar reason for the fourth equation. This eliminates the last four solutions out of the above eight listed, giving us 4 solutions total

Solution 3
Let
 . Then the given equation becomes
. Then the given equation becomes  .
.Let's set this equal to some value,
 .
.Clearing the denominator and simplifying, we get a quadratic in terms of
 :
:
Since
 and
and  are integers,
are integers,  is a rational number. This means that
is a rational number. This means that  is an integer.
is an integer.Let
 . Squaring and rearranging yields:
. Squaring and rearranging yields:
 .
.In order for both
 and
and  to be an integer,
to be an integer,  and
and  must both be odd or even. (This is easily proven using modular arithmetic.) In the case of this problem, both must be even. Let
must both be odd or even. (This is easily proven using modular arithmetic.) In the case of this problem, both must be even. Let  and
and  .
.Then:

 .
.Factoring 126, we get
 pairs of numbers:
pairs of numbers:  and
and  .
.Looking back at our equations for
 and
and  , we can solve for
, we can solve for  . Since
. Since  is an integer, there are only
is an integer, there are only  pairs of
pairs of  that work:
that work:  and
and  . This means that there are
. This means that there are  values of
values of  such that
such that  is an integer. But looking back at
is an integer. But looking back at  in terms of
in terms of  , we have
, we have  , meaning that there are
, meaning that there are  values of
values of  for every
for every  . Thus, the answer is
. Thus, the answer is  .
.Solution 4
Rewriting the expression over a common denominator yields
 . This expression must be equal to some integer
. This expression must be equal to some integer  .
.Thus,
 . Taking this
. Taking this  yields
yields  . Since
. Since  ,
,  . This implies that
. This implies that  so
so  .
.We can then take
 to get that
to get that  . Thus
. Thus  .
.However, taking
 ,
,  so
so  cannot equal 1.
cannot equal 1.Also, note that if
 ,
,  . Since
. Since  ,
,  will be an integer, but
will be an integer, but  will not be an integer since none of the possible values of
will not be an integer since none of the possible values of  are multiples of 9. Thus,
are multiples of 9. Thus,  cannot equal 9.
cannot equal 9.Thus, the only possible values of
 is 3, and
is 3, and  can be 1, 2, 7, or 14. This yields 4 possible solutions, so the answer is
can be 1, 2, 7, or 14. This yields 4 possible solutions, so the answer is  .
. - Let
 , and
, and  . Since
. Since  , we could let
, we could let  ,
,  , and
, and  . Now to get back to
. Now to get back to  we need another vertex
we need another vertex  . Now if we look at this configuration as if it was two dimensions, we would see a square missing a side if we don't draw
. Now if we look at this configuration as if it was two dimensions, we would see a square missing a side if we don't draw  . Now we can bend these three sides into an equilateral triangle, and the coordinates change:
. Now we can bend these three sides into an equilateral triangle, and the coordinates change:  ,
,  ,
,  ,
,  , and
, and  . Checking for all the requirements, they are all satisfied. Now we find the area of triangle
. Checking for all the requirements, they are all satisfied. Now we find the area of triangle  . The side lengths of this triangle are
. The side lengths of this triangle are  , which is an isosceles right triangle. Thus the area of it is
, which is an isosceles right triangle. Thus the area of it is  .
.
以上解析方式仅供参考
学术活动报名扫码了解!免费领取历年真题!
在线登记
最新发布
Raybet比分 课程体验,退费流程快速投诉邮箱: yuxi@linstitute.net 沪ICP备2023009024号-1